Lovegrove Mathematicals
"Dedicated to making Likelinesses the entity of prime interest"
Lovegrove Mathematicals
"Dedicated to making Likelinesses the entity of prime interest"
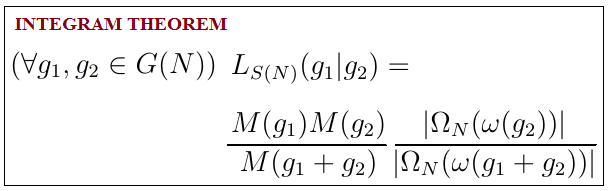
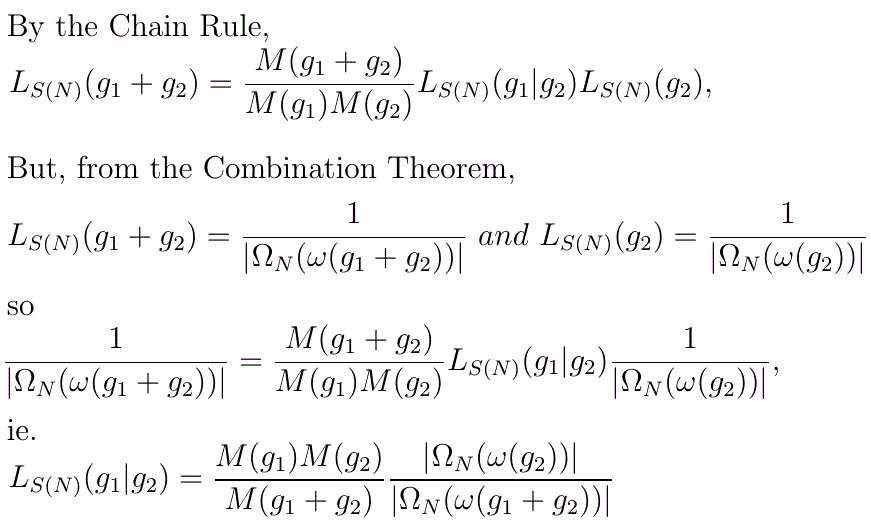
When ω(g1)=1, this reduces to Laplace's Law of Succession. If it is required to look more than one observation ahead, it is not possible to use the Law of Succession but the Integram Theorem can be used, instead.
A question, capable of being answered "Yes" or "No", is asked of 10 people. 7 reply "Yes" and 3 reply "No".
What is the Likeliness that
Represent "Yes" by "1" and "No" by "2". The underlying set is then S(2) and the given integram is g2=(7,3).
Part 1. The required integram is g1=(1,0), so we need LS(2)((1,0)|(7,3)). We are looking only one observation ahead, so the Law of Succession may be used.
We then get LS(2)((1,0)|(7,3))=(7+1)/(10+2)=8/12=2/3
Part 2. The required integram is g1=(2,0), so we need LS(2)((2,0)|(7,3)). We are looking two observations ahead, so the Law of Succession cannot be used. We shall use the Integram Theorem, instead.
We have g1+g2=(2,0)+(7,3)=(9,3), and so
Hence LS(2)((2,0)|(7,3))=(1.120/220).(11/13)=6/13
Note that (2/3)2=4/9 (=0.4444...), not 6/13 (=0.4615...), so it would be incorrect -when answering Part 2- to use the Law of Succession (as in Part 1) and then square the result: doing so would be equivalent to assuming that the best estimate of a square is the square of the best estimate, which is the same logical trap that the Perfect Cube Factory falls prey to.